Talk Prep

By | | , , , , , ,
Comments Off on Talk Prep

snapshot of pencil and paper with scribblings and sketches in boxesHow do I prepare my research talks? I usually just sit down with a pencil, some paper and a cup of something warm, and I just draw/map out the story. Each box is a beat of the narrative, and ends up corresponding to one or two slides (if I’m doing slides). Then I’m more or less done.

(The facility of this old school approach is that drawing it out keeps it visual, less heavy with equations. Too many (if any) slides or long periods laden with equations (at least in physics) just aren’t so great. Also, it allows me to move these thumbnails/pages/sketches around if I need to, to sculpt the narrative. I can sit back and see if it’s all there at the end.)

(For this Harvard/MIT seminar, scheduled for Wednesday, I don’t yet know if I am going to get to give it. Wisdom about travel and gatherings is a bit uncertain right now, and things are changing as I type. Decisions on Monday. Update:- we changed it to a remote talk.)

–cvj

Custard

By | |
Comments Off on Custard

When looking for an excuse to have some custard, simply whip up an upside down cake built on a bit of fruit, spices, and whatnot you might have (here: apples cooked in butter, basking on caramel, with toasted walnuts, cinnamon…etc).

–cvj

Synchronicity?

By | | , ,
3 Comments

Picture from my notes showing Bilbo, Ballin, and SmaugHere’s a striking coincidence. Last Friday I was preparing to deliver a lecture on special relativity to my undergrad General Relativity class with this Hobbity thought experiment (that helps one discover Lorentz-Fitzgerald contraction), when I heard that Christopher Tolkien (the boy the Hobbit was originally written for) had died. (RIP. And thanks for the maps, the Silmarillion and so much more.)

I took the opportunity in class to pay brief tribute to him and to encourage a new generation to delve into the books, the world, and more, as expanded and illuminated for us by Tolkien the younger.

-cvj

Black Holes and a Return to 2D Gravity! – Part II

By | | , , , ,
Comments Off on Black Holes and a Return to 2D Gravity! – Part II

(A somewhat more technical post follows.)

Continuing from part I: Well, I set the scene there, and so after that, a number of different ideas come together nicely. Let me list them:

illustration of JT gravity background

What “nearly” AdS_2 looks like via JT gravity. The boundary wiggles, but has fixed length 1/T.

  • Exact solution of the SYK model (or dual JT model) in that low temperature limit I mentioned before gave an answer for the partition function Z(\beta), by solving the Schwarzian dynamics for the wiggling boundary that I mentioned earlier. (The interior has a model of gravity on AdS_2, as I mentioned before, but as we’re in 2D, there’s no local dynamics associated with that part. But we’ll see in a moment that there’s very interesting stuff to take into account there too.) Anyway, the result for the Schwarzian dynamics can be written (see Stanford and Witten) in a way familiar from standard, say, statistical mechanics: Z_0(\beta)=\int dE \rho_0(E) \exp(-\beta E), where \rho_0(E)\sim\sinh(2\pi\sqrt{E}) is the spectral density of the model. I now need to explain why everything has a subscript 0 in it in the last sentence.
  • On the other hand, the JT gravity model organises itself as a very interesting topological sum that is important if we are doing quantum gravity. First, recall that we’re working in the “Euclidean” manner discussed before (i.e., time is a spatial parameter, and so 2D space can be tessellated in that nice Escher way). The point is that the Einstein-Hilbert action in 2D is a topological counting parameter (as mentioned before, there’s no dynamics!). The thing that is being counted is the Euler characteristic of the space: \chi=2-2g-b-c, where g,b,c are the number of handles, boundaries, and crosscaps the surface has, characterising its topology. Forget about crosscaps for now (that has to do with unorientable surfaces like a möbius strip (g=0,b=1,c=1) – we’ll stick with orientable surfaces here). The full JT gravity action therefore has just the thing one needs to keep track of the dynamics of the quantum theory, and the partition function (or other quantities that you might wish to compute) can be written as a sum of contributions from every possible topology. So one can write the JT partition function as Z(\beta)=\sum_{g=0}^\infty\hbar^{-(1-2g)}Z_g(\beta) where the parameter \hbar weights different genus surfaces. In that sum the weight of a surface is \hbar^{-\chi} and b=1 since there’s a boundary of length \beta, you may recall.

    The basic Schwarzian computation mentioned above therefore gives the leading piece of the partition function, i.e., g=0, and so that’s why I put the subscript 0 on it at the outset. A big question then is what is the result for JT gravity computed on all those other topologies?!

    • Click to continue reading this post →

Black Holes and a Return to 2D Gravity! – Part I

By | | , , , , ,
1 Comment

(A somewhat more technical post follows.)

Escher’s “Circle Limit III”, nicely illustrating (Euclidean) AdS_2 for us.

Well, I think I promised to say a bit more about what I’ve been up to in that work that resulted in the paper I talked about in an earlier post. The title of my paper, “Non-perturbative JT gravity” has JT (Jackiw-Teitelbiom) gravity in it, so I could start there.

JT gravity is a model of two dimensional gravity that goes back many decades. But there’s not much going on in 2D (or (1+1) dimensions, one space and one time), I hear you cry! Well, nevertheless this turns out to be a very useful arena for studying very important quantum key properties of black holes in more realistic dimensions. Let me take a step back to unpack that.

There is a long tradition (which you might know about) of studying models of black holes in string/M-theory, in different limits and approaches, each with their own advantages and disadvantages. The simplest models are often too simple to capture some of the important features of black holes, and some models get nicely at certain aspects while having little to say about others. And of course, some of the more realistic (but still simple) black hole models are still too complicated to be directly solvable in order to reliably explore phenomena of interest.

I’d say that a certain class of models that has been discussed a lot recently has something new to offer. The prototype is something called the SYK model (Sachdev-Ye-Kitaev). It’s an even more crazy sounding starting point than 2D gravity, as it is a 1D model. There’s no space at all, just time: It is a model in (0+1) dimensions, if you like. It’s a special model of quantum mechanics, actually a bunch of 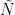\tilde N fermions (where \tilde N is large), with certain random couplings between them. It was noticed that this model, at temperature T, as a simple thermal with quantum chaos, scrambles quantum information at a particular rate (measured by the “Lyapunov exponent” \lambda_L) that was associated with the scrambling rate of black holes: \lambda_L=2\pi k_B T/\hbar. So it became of interest to study it as a simple, solvable model of this sort of behaviour. (Kitaev made a convincing case in a series of talks at the KITP back in 2015 that if you find a model with black-hole-like scrambling, it has a secret dual gravitational character to it, in a sense we’ve discussed here on the blog before. – I recommend looking at the talks (here and here), and also a nice paper of Maldacena, Shenker, and Stanford (also from Spring 2015) that discusses the gravitationally-motivated bounds on chaos that the SYK model saturates.).

Well, I heard people mention the SYK model increasingly over 2015 but I was thinking about too many other things (and was mostly dealing with being mostly sleep-deprived as a new dad), and so did not really pay much attention. Bandwidth issues, we’d say these days. I was happy to declare “we’ve got some of our best people on it”, and continued chipping away on other things where I could. Then in December 2017 I found myself sitting next to David Gross on a flight to the East coast (long story I forgot to blog about), and he mentioned that he’d been really excited by the SYK model and maybe I should have a look, since there’s a lot there that I’d probably like. And *still* some time went by without me clearing up bandwidth to look at any of it.

Catch up began in the Fall of last year (2019), but only after similarly missing following what was going on in a related area: JT gravity. One way of thinking about how JT gravity enters the story is to simply state that it is a holographic dual of the SYK model, in the sense of AdS/CFT, where you have a gravity theory on one side, and it is dual to a (conformally invariant) non-gravitational field theory on the other side. The key thing is that the gravitational theory has one dimension more than the field theory. I’ve spoken of such things a lot here on this blog so I won’t review, but instead let you dig a bit and find things to read in the archives. (You could put AdS/CFT in the search window, or maybe start here.)

A ball showing the AdS/CFT setup. The interior has gravity on an anti-de Sitter spacetime, the the boundary has a dual (conformal) field theory.

Simply/glibly put, the duality says that some complicated (strongly coupled) dynamics of a field theory can be re-written as a simpler dynamics involving gravity, and the “re-writing” uses an additional spatial dimension. Most famously you have (super) Yang-Mills theory in 4D (which is conformally invariant, so let’s write it as CFT_4) being equivalent to a theory of gravity in 5D anti-de Sitter (AdS_5). So AdS_5/CFT_4. Many examples are known in various dimensions that fill out the pattern AdS_(d+1)/CFT_d. The picture I like to draw is of a ball (see above right), the interior of which is the AdS space where gravity is operating (I’ve cut a hole for you to see the inside), and the boundary of which has the (one dimension fewer) conformal field theory. You often hear people refer to the “boundary” and the “bulk” – well, that’s the picture.

Important technical note: In the setup I drew above, the metric which measures spatial distances makes spacings smaller and smaller as you move out from the Click to continue reading this post →

More Pie

By | |
Comments Off on More Pie

Well, you know the saying: When life hands you several apples, some blackberries, and a chunk of pastry left over from your last pie-making… you make another apple-blackberry pie, taking the opportunity to make it even better!

multi-panel picture of apple blueberry pie being made, and finished product.

(No? Never heard that saying? Huh.)

-cvj

Chalky

By | | ,
Comments Off on Chalky


I realized recently that I’ve forgotten a great deal of my drawing skills, settling back into some clunky habits, due to zero practice. But I’m going to need them back for a project, and so will start teaching myself again. Above is a (digital) chalk doodle I did yesterday.

–cvj

Rolling…

By | |
Comments Off on Rolling…

And so it began… All this butter had to end up inside this little bit of flour/water mix. But in lots of tasty layers of deliciousness! (And I’ll do this four times, and so that’s my morning.)

More later. I love puff pastry!

–cvj

Spectral (again)

By | | , ,
6 Comments

A newly computed spectral density function for a new example…

Oh, this? I’m very pleased with it. I’ll tell you more about what it is at a later point, but this is mostly for my own entertainment. It’s a sort of big brother to the spectral curve shown in the previous post. I’d been thinking about how to get this curve (those plus marks) to come out right for a few days, and repeatedly getting it not quite right*, with puzzlement and frustration. And then while out on a run it came to me how to do it right…. Then after writing the matlab routines to make my solution happen, out it popped! Hurrah. (Update: The inset was added to show more detail. Now it is in the paper as an added extra.)

(* Update: “not quite right” means, for example, that the large E behaviour should have been E^(3/2), generalising the E^(1/2) of the previous example, but it wasn’t for a bit, because of a normalization issue. All fixed now!)

To (most of) you it’s just a bunch of dots and wiggles and that’s ok. To me is some very nice physics that connects things I’d been thinking about back some 30 years ago with things I’m thinking about now. More later. Sorry if this is annoying, I’m just very pleased with the result.

-cvj

Spectral

By | | ,
7 Comments

Oh, this was a recent post I did on social media that I think ought to go here too. I will try to write a longer post about the physics behind this (in involves black holes, quantum gravity, string theory, quantum chaos, and a host of other things):

A spectral density function of particular interest to me and a recent project on 2D quantum gravity.

Were you looking for more reasons to read my recent paper on JT gravity? This figure from it is my favorite reason. It summarizes a lot of the abstract – identical perturbation theory (right) but better non-perturbative regime (left). Go! https://arxiv.org/abs/1912.03637

-cvj

A Return

By | | , ,
Comments Off on A Return

Panorama showing snow on the mountains and hills near Los Angeles. (Click for larger view. Taken 30th November 2019)

It has been two weeks since it began, but I’m still pleased with the arrival of relatively chilly weather here in LA, because the heat, dust, grime, and smoke of the Summer and into the Fall (from fires, endless hot days, etc.,) was seemingly relentless, and really getting me down. The panorama above (click for larger view), taken from the top of Mount Hollywood, shows the return of snow to the mountains and nearby hills as a result of some of that chill (this meant some heavy rain in the actual city, which was nice.)

Speaking of returns, I’m back! You’ll have noticed that I’ve been bit quiet here on the blog, for which I apologise. I hope that you’ve been following my (quite regular) microblogging on twitter, instagram and Facebook, however – It is not the same as some of the longer in-depth posts I do here, but it might still help you keep in touch, so do follow on those platforms if you wish. Links in the sidebar.

This semester has been a very busy one, in which I’ve felt rather pulled apart. New routines due to various things too tedious to mention have meant that the places in my schedule where I might usually find time to do a longer thoughtful blog post were harder to come by, and by later in the evening when I’d time to take a breather, it would be the wrong part of the day to pull together coherent and interesting thoughts. But I am working back to a new approach and/or routine where there is some time for that. This includes the fact that it has occurred to me that since I use dictation more in place of lots of my typing (particularly on smaller devices like phones, iPads and watches), I might start banking snippets of thoughts when I can, using dictation, and then edit and concatenate later. We shall see if that helps me create more, er, “content”. Either way, do look out for posts in the coming days and weeks.

I’ve actually a LOT of things to tell, since I’ve been doing a lot – fascinating meetings and conferences, and research projects of various sorts. So it isn’t for lack of material that I’ve not said much since August, but quite the opposite. More to come!

-cvj

Two Days at San Diego Comic-Con 2019

By | | , , , , , ,
Comments Off on Two Days at San Diego Comic-Con 2019

Avengers cosplayers in the audience of the Friday panel.

It might surprise you to know just how much science gets into the mix at Comic-Con. This never makes it to the news of course – instead its all stories about people dressing up in costumes, and of course features about big movie and TV announcements. Somewhere inside this legendary pop culture maelstrom there’s something for nearly everyone, and that includes science. Which is as it should be. Here’s a look at two days I spent there. [I took some photos! (All except two here – You can click on any photo to enlarge it.]

Day 1 – Friday

I finalized my schedule rather late, and so wasn’t sure of my hotel needs until it was far too late to find two nights in a decent hotel within walking distance of the San Diego Convention Center — well, not for prices that would fit with a typical scientist’s budget. So, I’m staying in a motel that’s about 20 minutes away from the venue if I jump into a Lyft.

My first meeting is over brunch at the Broken Yolk at 10:30am, with my fellow panellists for the panel at noon, “Entertaining Science: The Real, Fake, and Sometimes Ridiculous Ways Science Is Used in Film and TV”. They are Donna J. Nelson, chemist and science advisor for the TV show Breaking Bad (she has a book about it), Rebecca Thompson, Physicist and author of a new book about the science of Game of Thrones, and our moderator Rick Loverd, the director of the Science and Entertainment Exchange, an organization set up by the National Academy of Sciences. I’m on the panel also as an author (I wrote and drew a non-fiction graphic novel about science called The Dialogues). My book isn’t connected to a TV show, but I’ve worked on many TV shows and movies as a science advisor, and so this rounds out the panel. All our books are from Click to continue reading this post →

News from the Front XIX: A-Masing de Sitter

By | | , , , , ,
Comments Off on News from the Front XIX: A-Masing de Sitter

Diamond maser. Image from Jonathan Breeze, Imperial College

This is part 2 of a chat about some recent thoughts and results I had about de Sitter black holes, reported in this arxiv preprint. Part 1 is here, so maybe best to read that first. (Note: I’ve made some updates because I’ve refined the physics.)

Now let us turn to de Sitter black holes. I mean here any black hole for which the asymptotic spacetime is de Sitter spacetime, which is to say it has positive cosmological constant. This is of course also interesting since one of the most natural (to some minds) possible explanations for the accelerating expansion of our universe is a cosmological constant, so maybe all black holes in our universe are de Sitter black holes in some sense. This is also interesting because you often read here about explorations of physics involving negative cosmological constant, so this is a big change!

One of the things people find puzzling about applying the standard black hole thermodynamics is that there are two places where the standard techniques tell you there should be a temperature associated with them. There’s the black hole horizon itself, and there’s also the cosmological horizon. These each have temperature, and they are not necessarily the same. For the Schwarzschild-de Sitter black hole, for example, (so, no spins or charges… just a mass with an horizon associated with it, like in flat space), the black hole’s temperature is always larger than that of the cosmological horizon. In fact, it runs from very large (where the black hole is small) all the way (as the black hole grows) to zero, where the two horizons coincide.

You might wonder, as many have, how to make sense of the two temperatures. This cannot, for a start, be an equilibrium thermodynamics system. Should there be dynamics where the two temperatures try to equalise? Is there heat flow from one horizon to another, perhaps? Maybe there’s some missing ingredient needed to make sense of this – do we have any right to be writing down temperatures (an equilibrium thermodynamics concept, really) when the system is not in equilibrium? (Actually, you could ask that about Schwarzschild in flat space – you compute the temperature and then discover that it depends upon the mass in such a way that the system wants to move to a different temperature. But I digress.)

The point of my recent work is that it is entirely within the realm of physics we have to hand to make sense of this. The simple system described in the previous post – the three level maser – has certain key interconnected features that seem relevant:

  • admits two distinct temperatures and
  • a maximum energy, and
  • a natural instability (population inversion) and a channel for doing work – the maser output.

My point is that these features are all present for de Sitter black holes too, starting with the two temperatures. But you won’t see the rest by staring at just the Schwarzschild case, you need to add rotation, or charge (or both). As we shall see, the ability to reduce angular momentum, or to reduce charge, will be the work channel. I’ll come back to the maximum Click to continue reading this post →